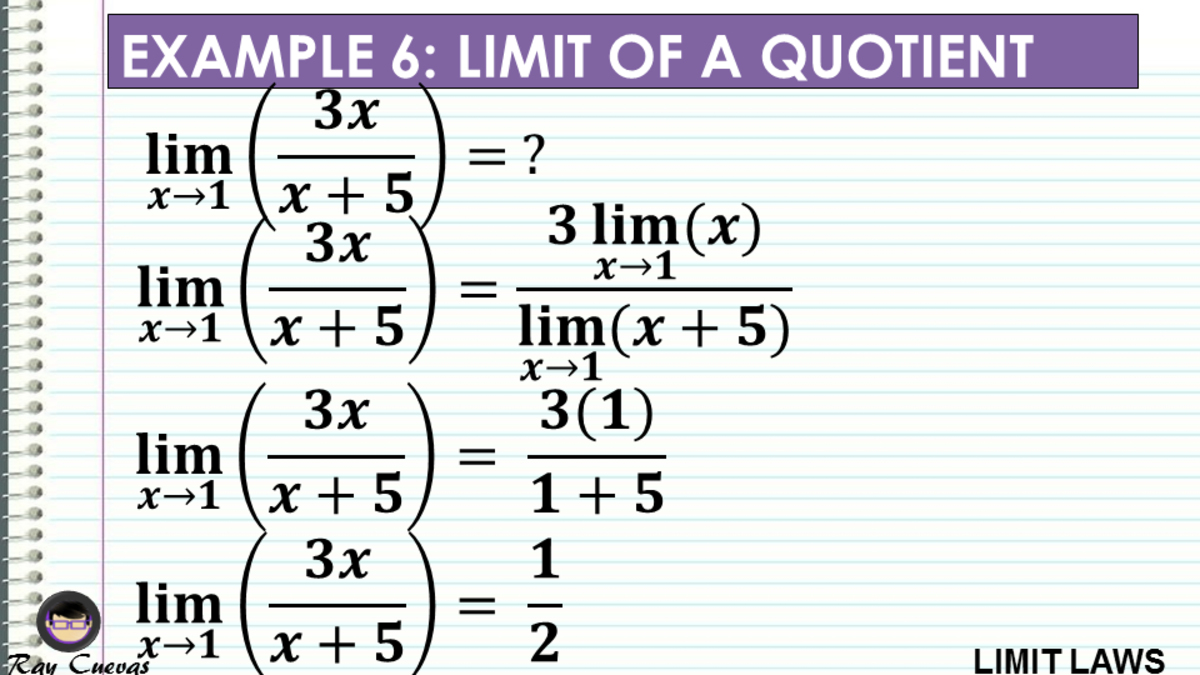Glory Info About How Do You Change A Variable In Limits Excel Graph Shade Area Between Lines

Suppose a change of variables \(x = x(s,t,u)\text{,}\) \(y = y(s,t,u)\text{,}\) and \(z = z(s,t,u)\) transforms a closed and.

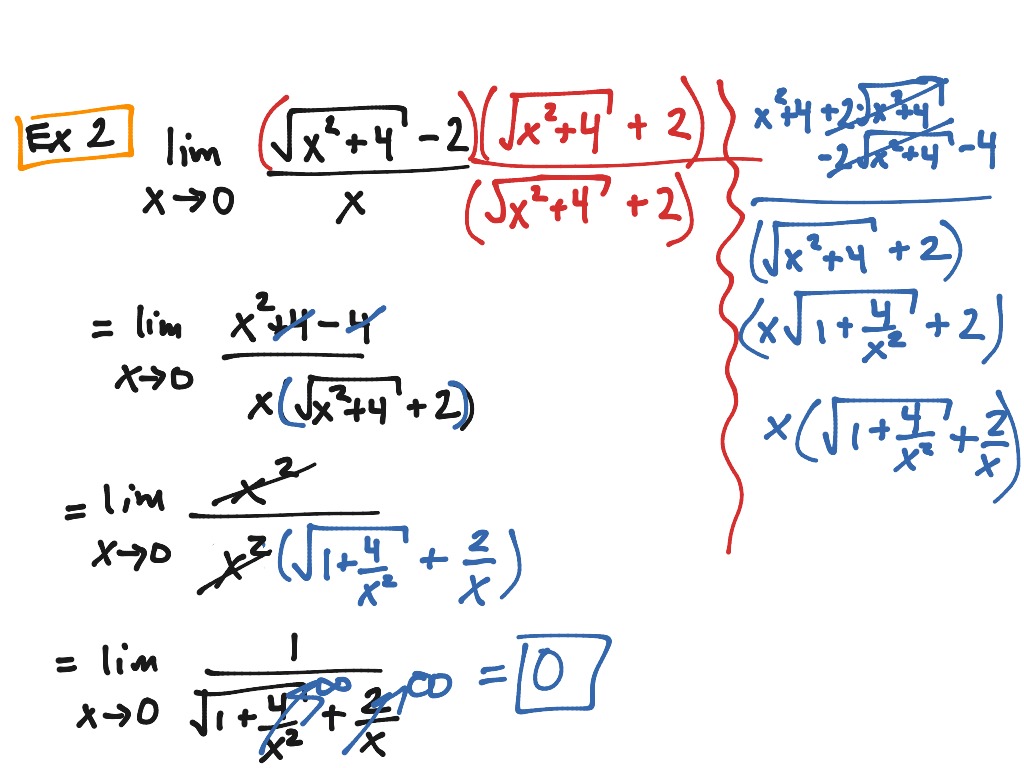
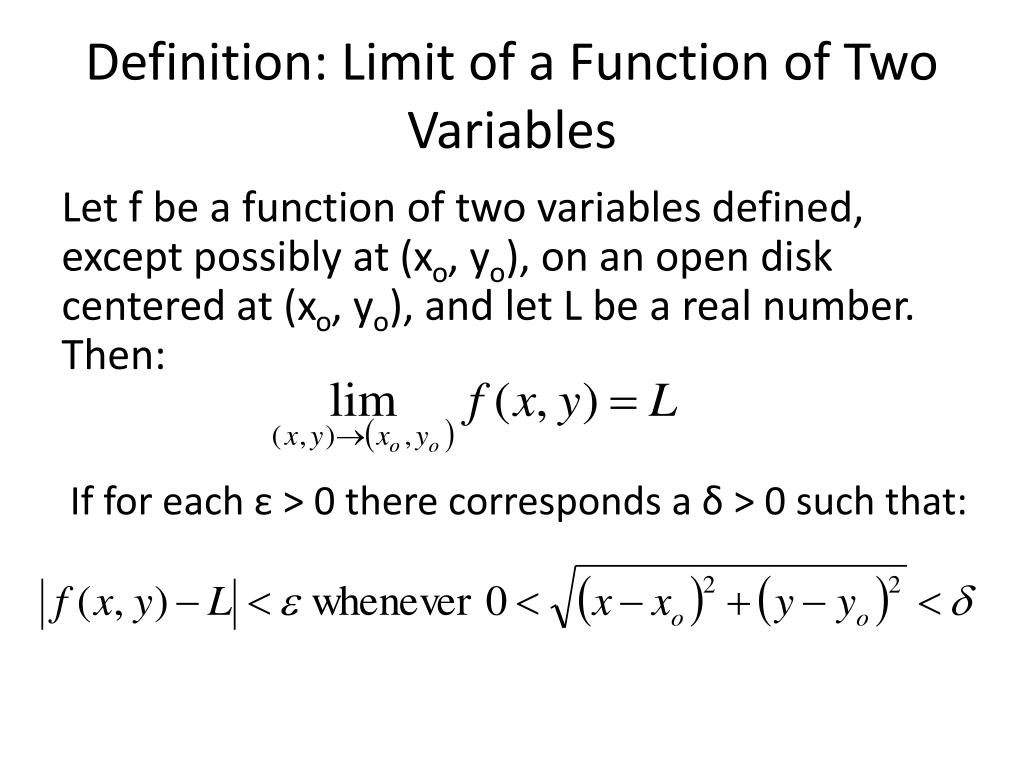
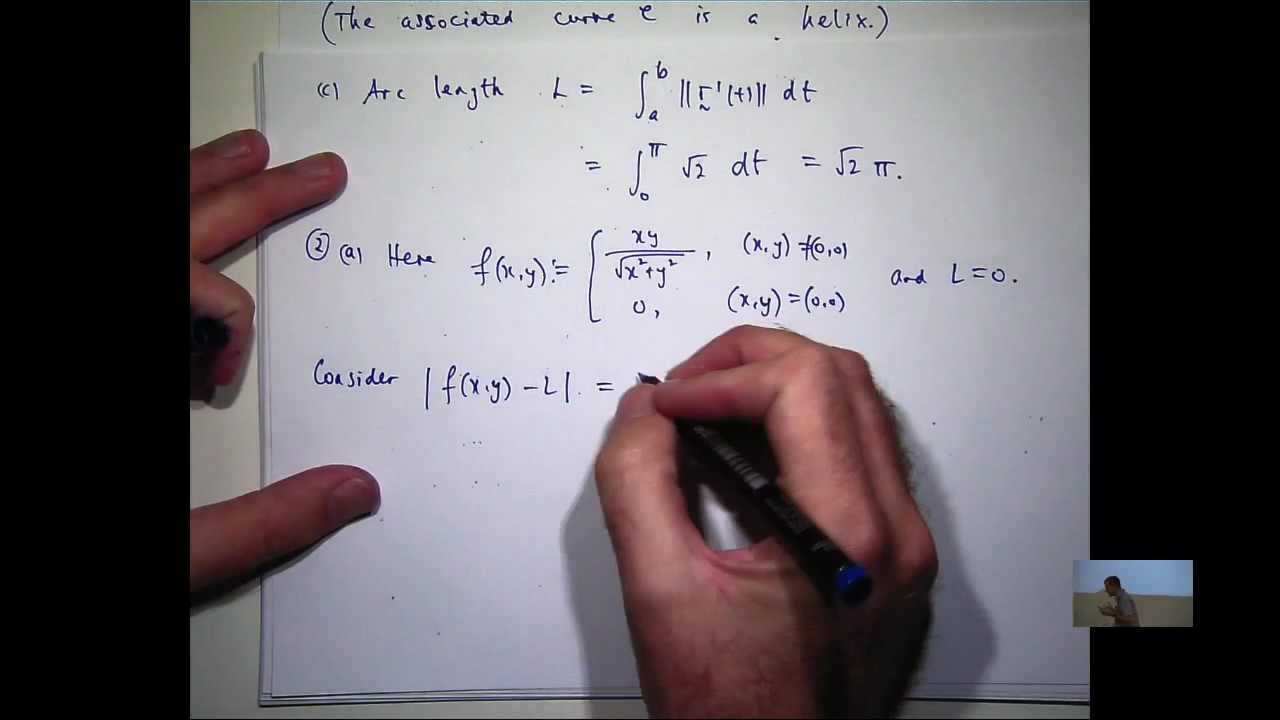
How do you change a variable in limits. In this note we examine how to make a “change of variables” or a “substitution” when calculating limits. Do i use math.max methods here too? In fact, we will concentrate mostly on limits of functions of two variables,.

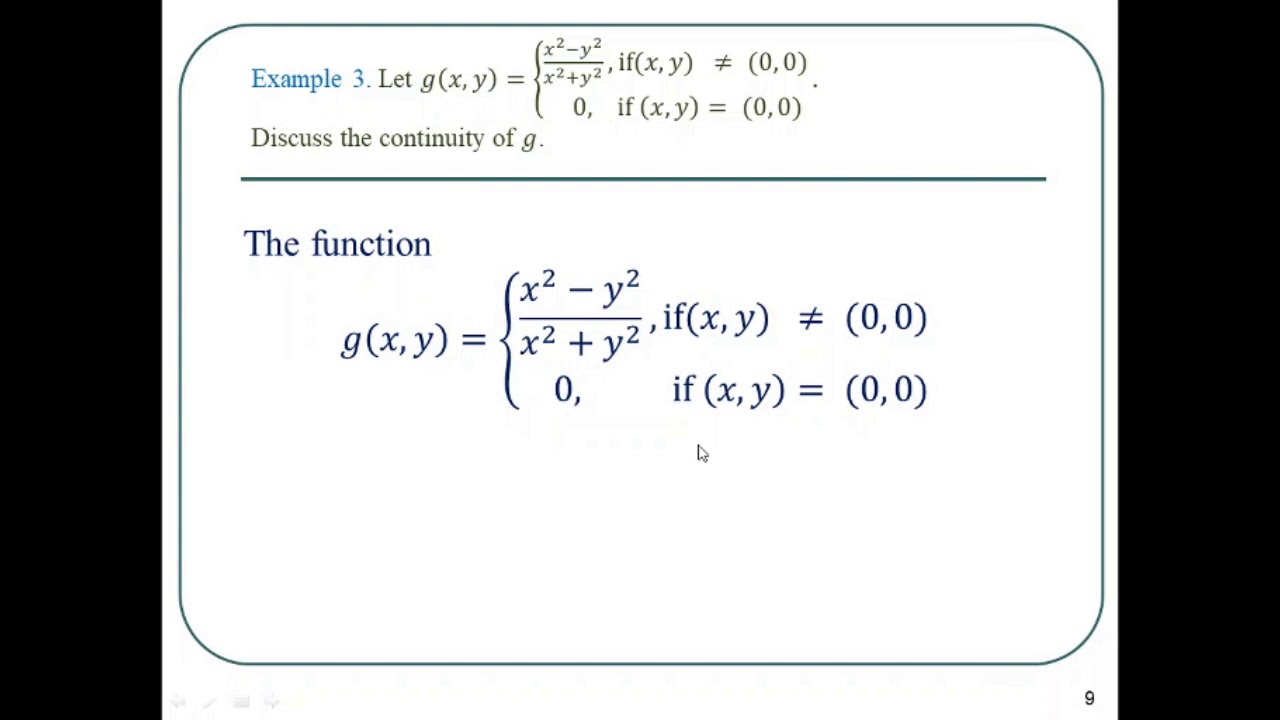
The example that triggered me is the limit you obtain while differentiating $e^x$: Right click them as they appear in the project canvas and click edit. In this section we will take a look at limits involving functions of more than one variable.

It will let you set the min and max values. How to use a change of variable to find a limit corresponding to the indeterminacy 0/0 for a quotient of polynomials. $$ so what we have is an inner function $g(t) = \tan t$.

For reference, see the 5.5 manual, the 5.6 manual and @quassnoi's answer If not, it will print you lose and break the whole loop, else continue. There we simply change the.

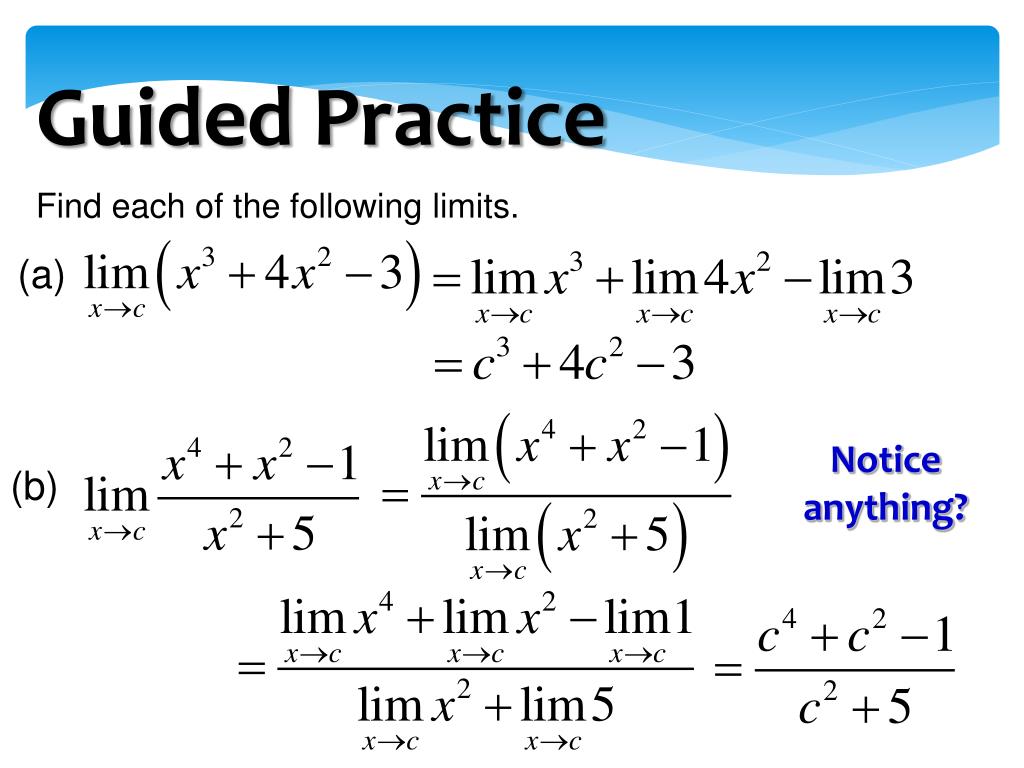
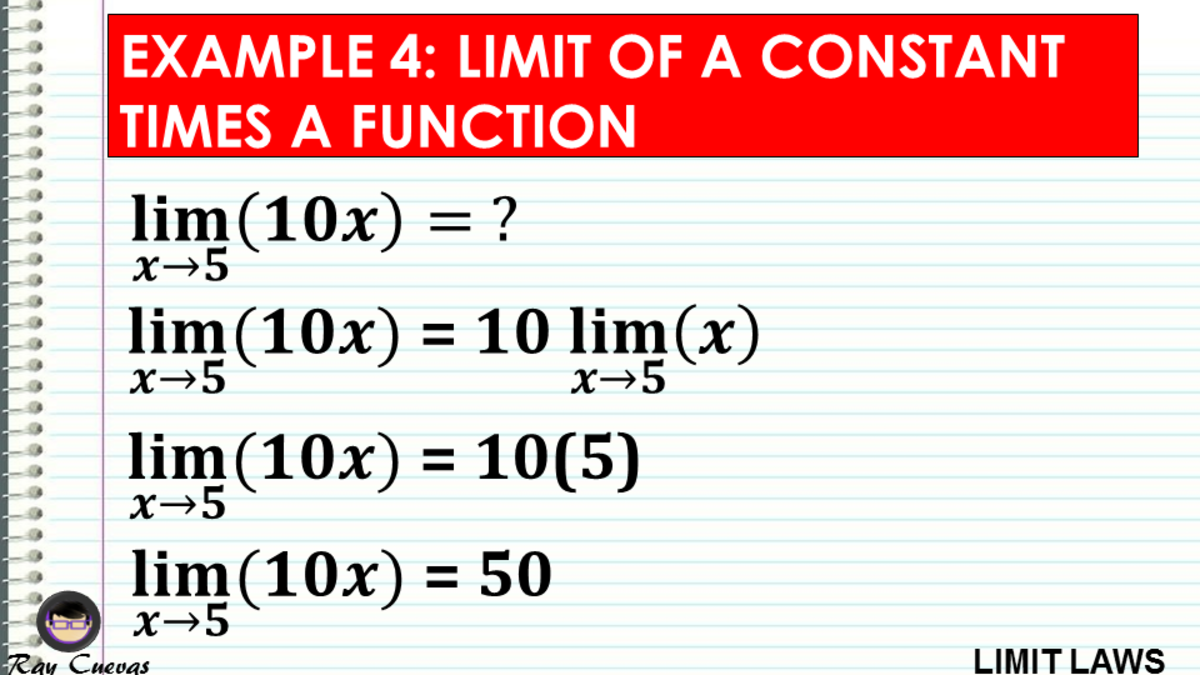
Use the limit laws to evaluate the. This change of variables often makes any. As of mysql version 5.5.6, you can specify limit and offset with variables / parameters.

It's important to know all these techniques, but it's also important to know when to apply which. You can add a condition inside the for loop which checks if the number is <= 21 or not. In the following theorem, we assume that the substitution function u =.

When we use the substitutions \(x = g(u,v)\) and \(y = h(u,v)\) and then change the limits of integration accordingly. The limits of $y = \int_{\sigma_0}^{r_m} \frac{dr}{r} $ Recognize the basic limit laws.

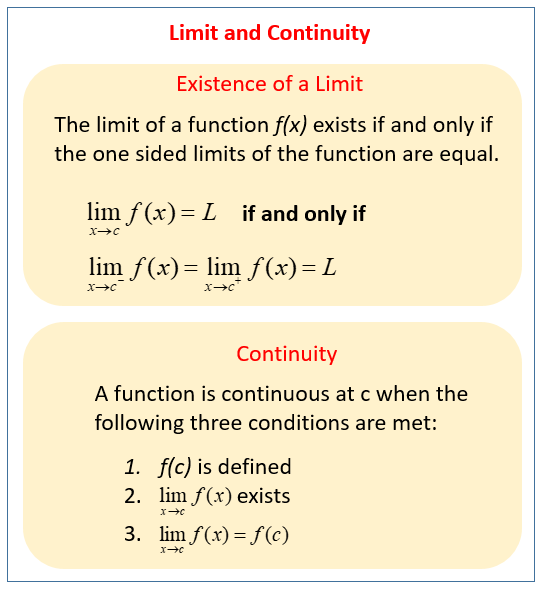
Theorem of change of variables in limits: Use the limit laws to evaluate the limit of a function. There are many techniques for finding limits that apply in various conditions.

Try simplifying the function with a change of variable! Evaluate the limit of a function by factoring. Basically we're changing/substituting $g(x)$ with $y$.

When we do substitution in a limit, we have to change all appearances of the original variable, which also includes the place under lim. Change of variables in a triple integral. Read this lesson to see examples of the change of variables method for finding the limit of a function.