Nice Info About Is A Parabolic Curve Exponential Metric Line Chart

For example, for linear curves, i can choose two points on the curve and check if the midpoint is also on the curve.

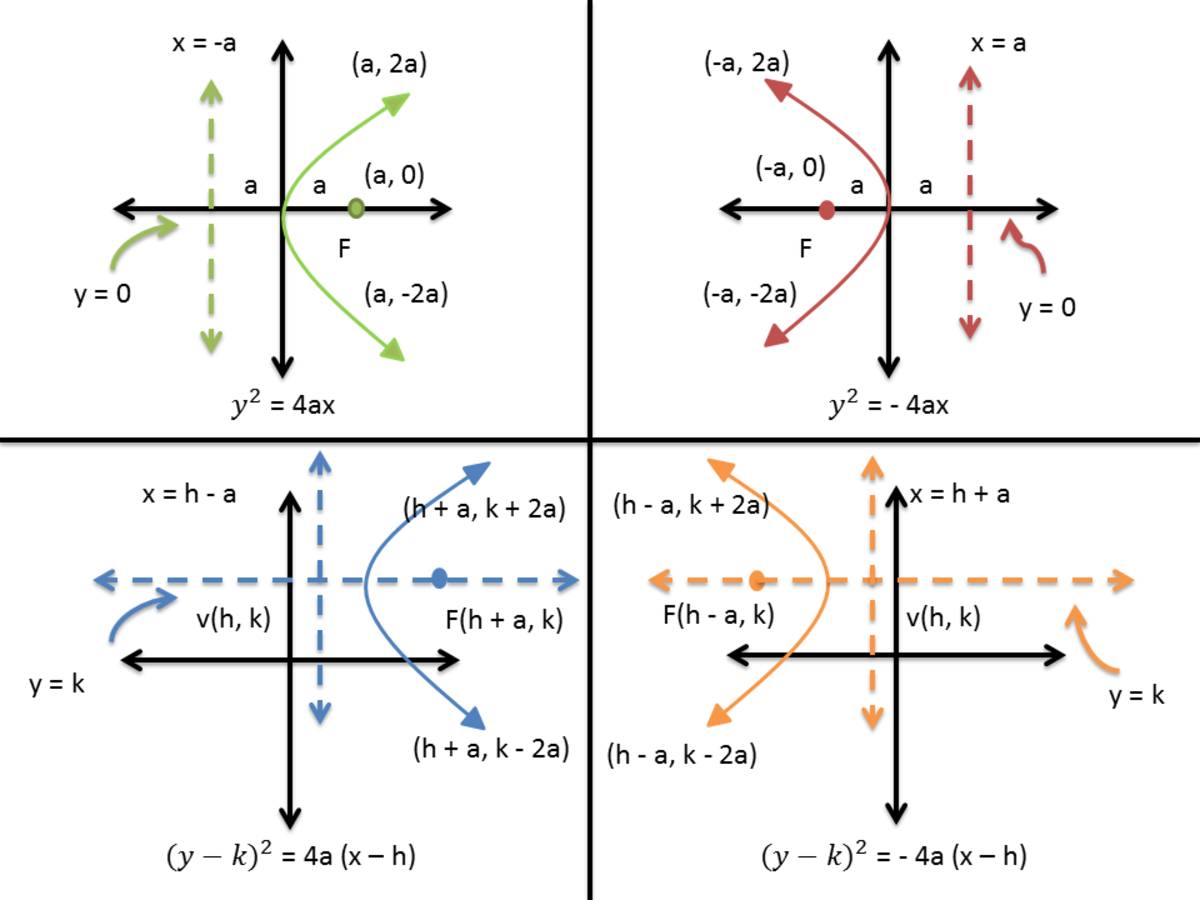
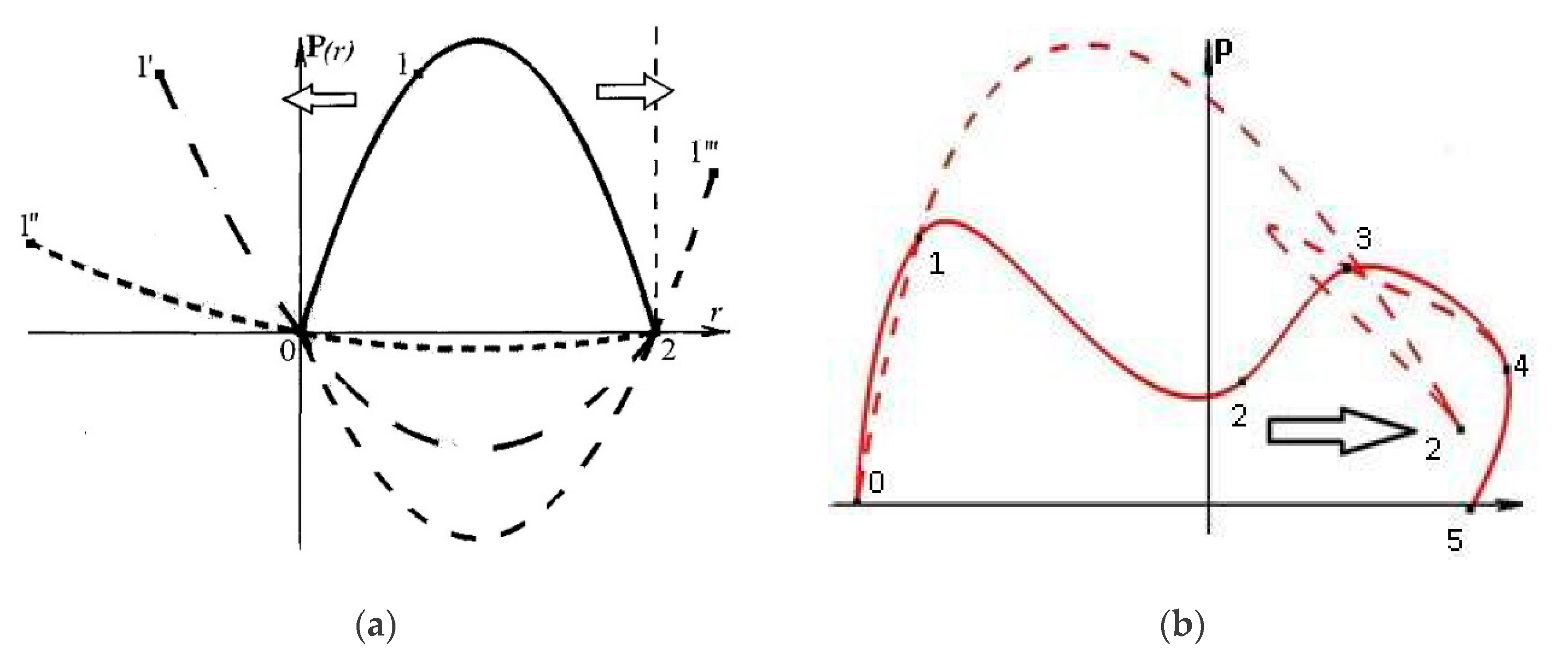
Is a parabolic curve exponential. This curve is a parabola. Its general equation is of the form y^2 = 4ax (if it opens left/right) or of the form x^2 = 4ay (if it opens up/down) A parabola refers to an equation of a curve, such that a point on the curve is equidistant from a fixed point and a fixed line.

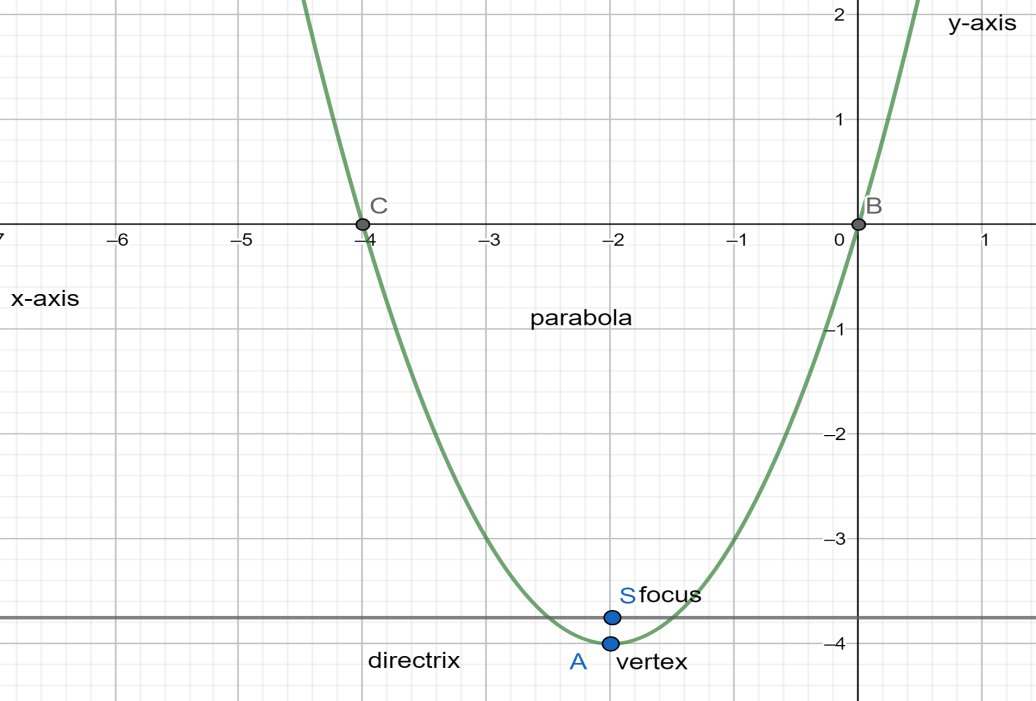
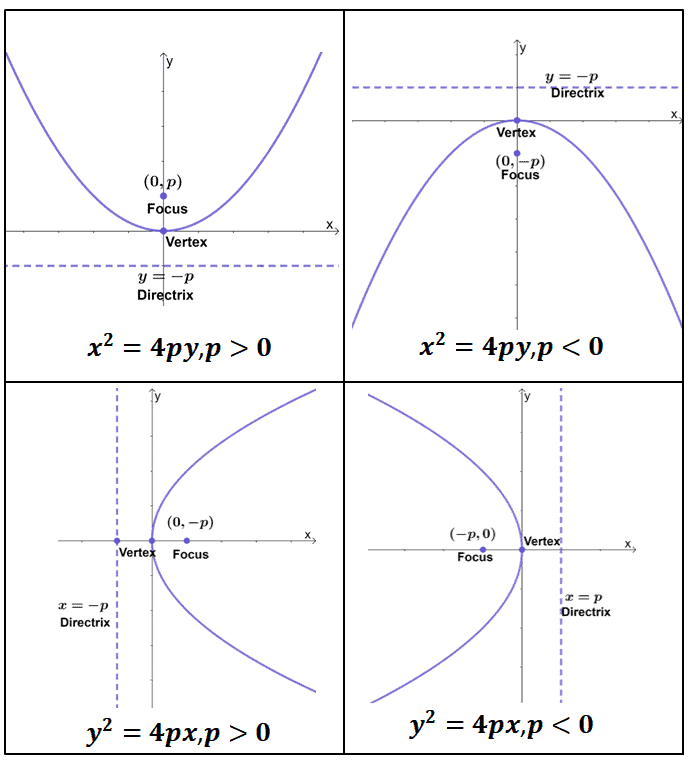
We’ll use the function observe how the output values in (figure) change as the input increases by. Like the ellipse and hyperbola, the parabola can also be defined by a set of points in the coordinate plane. The fixed point is called the focus, and the fixed line is called the directrix of the parabola.

Rest assured, the shape of the curve is parabolic. The axis of symmetry is the vertical line passing through the vertex. As a plane curve, it may be defined as the path (locus) of a point moving so that its distance from a fixed line (the directrix) is equal to its distance from a fixed point (the focus).

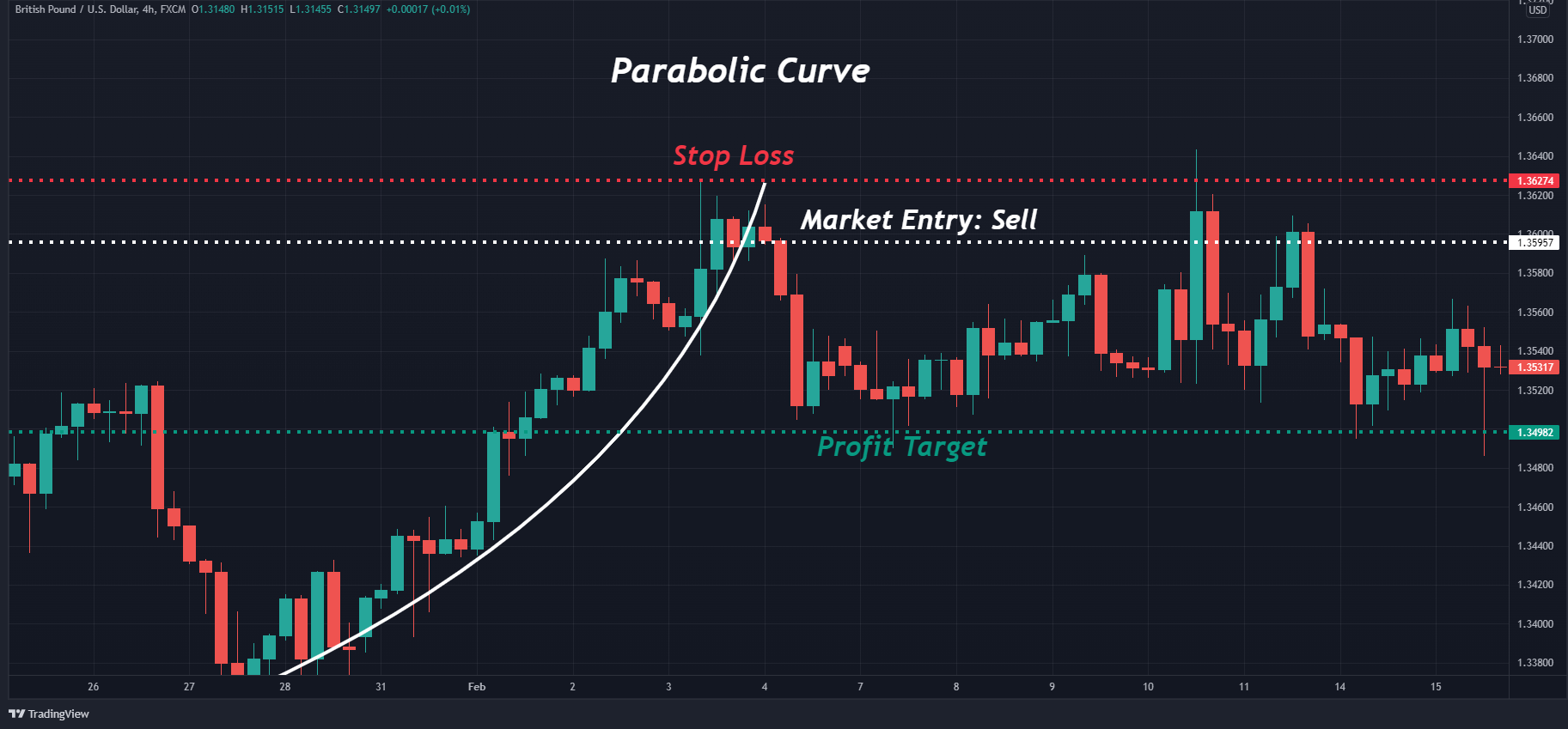
Get a piece of paper, draw a straight line on it, then make a big dot for the focus (not on the line!). The logarithmic curve looks a little like a portion of the downward opening parabola, but it never reaches a maximum as does the parabola, increasing, however, more and more slowly with increasing x. Graph vertical parabolas.

If $f(x) = e^x$, $f = f' = f'' =.$ and the derivative of any order is positive and continuous at any real number. Now we extend the discussion to include other key features of the parabola.

One description of a parabola involves a point (the focus) and a line (the directrix ). Parabola, open curve, a conic section produced by the intersection of a right circular cone and a plane parallel to an element of the cone. The graph of parabola is upward (or opens up) when the value of a.

For parabolas, i can examine the geometric relationship between the tangent at a point and the secant connecting the. These functions are so darn good at making hyperbolas that they're typecast for that role. The exponential function is a good example:

In geometrical terms, the parabola corresponds to the edge of slice of an inverted cone; A parabola is a particular type of geometrical curve which, algebraically, corresponds to a quadratic equation. The equation is y = a + bx + cx2 and the normal equations are.

The graph of the parabola is downward (or opens down), when the value of a is less than 0, a < 0. In quadratic functions, we learned about a parabola’s vertex and axis of symmetry. Solve applied problems involving parabolas.

For instance, just as the quadratic function maintains its parabolic shape when shifted, reflected, stretched, or compressed, the exponential function also maintains its general shape regardless of the transformations applied. What are the hyperbolic functions ( cosh and sinh )? It is hard to see the shape because of the window settings.